The Optical Design of Gemstones

There is new excitement in the gem-stone industry. Today, for the first time, scientific advances are allowing the detailed optical design, precision cutting and characterization metrology of beautiful jewels. Understanding how to cut a stone to best disperse and break light into a plurality of colorful scintillations has consistently been a major focus of the gemstone industry. Diamonds, for example, are marketed on the basis of the Four C’s: cut, color, clarity and carat weight. Of these four attributes, "cut" is the least understood by the general public and by jewelry industry professionals. The word is used to refer not only to the shape of the diamond but also to its proportions, symmetry and polish. The quality of the cut is primarily responsible for making a stone appear brilliant and colorful, or dull and lifeless. It is obvious that an understanding of how a gem manipulates light to produce the effects of brilliance (brightness), fire (dispersion) and scintillation (sparkle) is critical to the proper design of jewels.
Another important reason to understand the behavior of light in gemstones is to establish and apply grading systems.* Given the large variety of gem materials and shapes available today, grading systems that make use of metrics based on light response and beauty have been introduced. The gem-buying public also benefits from an understanding of light propagation in gemstones. The main focus of this article is to describe the role of optics in gem-stone design along with related trends in the gemstone industry.
* Professional organizations such as the American Gem Society (AGS) offer their members services that range from certifying the authenticity of gems to giving an expert opinion on quality.
The complexities of cut
By trial and error, ancient jewelers produced cuts that refracted light to give illumination life to gemstones. One of the first thought-provoking studies on how to cut gems was published by M. Tolkowsky1 in 1919. He systematically analyzed the optics of a diamond and estimated the best proportions for cutting
brilliant round diamonds. With minor changes, today’s standards for “ideal-cut” diamonds are based on Tolkowsky’s definitions.
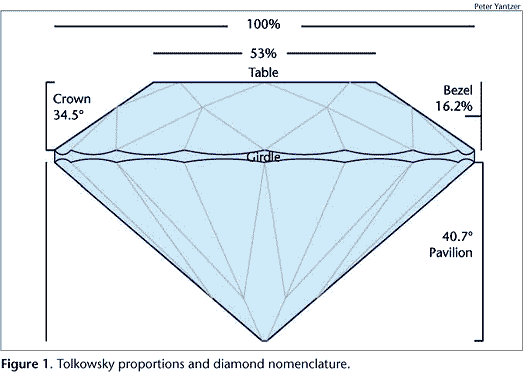
The round brilliant diamond shown in cross section in Fig. 1 is the best understood of all the shapes in which diamonds are cut. It consists of 57 facets, 33 in the crown (one for the crown table and 32 for the crown bezel) and 24 in the pavilion. Light enters the stone through the crown and is internally reflected until it is finally refracted and directed to the examiner’s eye or elsewhere. It is undesirable to have light entering or leaking out through the pavilion or girdle because it will reduce the potential of a diamond to show its brilliance. Thus the optical function of a stone’s cut is to bring light to the observer’s eye from above the crown. In performing this function, light is dispersed and colorful flashes — known in the gemstone industry as fire — can be observed. An additional objective is to create a cut by means of which a plurality of the stone’s facets are lit and others left dark, which produces a bright appearance on the crown known as brilliance.2 Illumination conditions and cut proportions determine whether fire or brilliance predominates. Scintillation, another attribute of gemstones, refers to their ability to produce an appearance of flashes of light when gemstone, observer or light source is moved. Gemstone beauty is a complex subject that involves the human visual system, stone cut proportions and illumination conditions. Putting technical considerations aside for the moment, it is indeed breathtaking to behold the beauty of a diamond that has been optimally cut.
Metrics for beauty
Recently, members of the gemstone industry have engaged in efforts to produce metrics for a diamond’s beauty that measure appearance attributes such as brilliance, fire and scintillation. Notable are the studies by the Gemological Institute of America (GIA)3,4 and a group of professionals at Moscow State University.5,6 To identify and quantify which cuts produce the best appearance, as well as to contribute to the establishment of a system to grade gems, the two organizations have independently defined metrics and made parametric studies of the proportions of the round brilliant diamond. It is well known, for example, that a decrease in the angle of the pavilion can be compensated by an increase in the angle of the crown. This concept is similar to that of lens bending in lens design. It should be noted, in any case, that for fancy-shape cut gem-stones — such as the marquise, oval and heart — the understanding of light propagation and its link to optimal design is still somewhat of an open question. Figure 3. (a) Representation of the angular spectrum, (b) integrated angular spectrum (relative collected energy vs. angle), (c) change of integrated angular spectrum with color. Figure 4. (a) Effect of facet illumination in producing contrast, (b) multiple light sources accentuating fire. James Caudill (a) (b) to the gem and collected on the hemisphere.
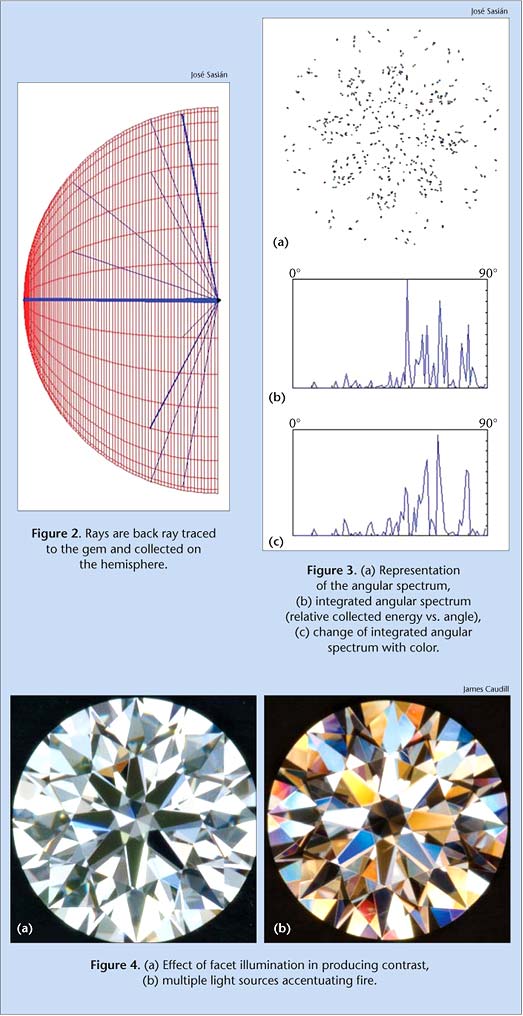
One insightful approach to analyzing light propagation in gems is to use the concept of geometrical angular spectrum. Here angular spectrum refers to the set of ray-angle directions that can make a gem’s facet bright. Rather than forward illuminating a stone, a reverse ray trace indicates the ray directions that can actually bring light to the observer’s eye (see Fig. 2). If these directions are projected into a hemisphere (as described by Meinel and Meinel 7 ) and centered on the gem, a two-dimensional map can be obtained that shows the directions or angles that can contribute to brilliance and fire [see Fig. 3(a)]. The observer’s head can block some of the central angles, so one goal of the optical design of gems is to control how much angular range can be blocked in this way. Some diamonds, called “nail heads,” lack brilliance because the pavilion has nearly a 45° angle that makes the stone act as a retroreflector and prevents ambient lighting from reaching the observer’s eye. The summation of the ray energy over concentric rings that are equally spaced on the hemisphere permits quantification of the angular spectrum [see Fig. 3(b)]. For the Tolkowsky cut — 34.5° crown angle, 40.7° pavilion angle, 53% table-to-diameter ratio — which has become a standard, about 75% of the energy reaches the hemisphere, about 17% corresponds to reflection on the crown which is not taken into consideration because it represents glare and about 9% is light leakage through the pavilion. In the hemisphere, high angles are from 76° to 90° (observer head), medium angles are from 45° to 75° and low angles are from 0° to 44°. In the Tolkowsky cut, about 15% of the energy is directed to the high angles, 51% to the medium and 8% to the low angles. Other cut proportions produce different angular spectrums and have different light proportions in the low, medium and high angles. An angular spectrum can be considered a gem signature because it intrinsically carries the cut proportions. The change of angular spectrum with color (blue and red) gives a measure of the dispersion and ray directions that can produce fire [see Fig. 3(c)]. When a stone is tilted, the angular spectrum increases in the low angles and the energy that reaches the hemisphere decreases. The directions that can produce fire are increased and fire may decrease toward the low angles as well. This suggests that for the analysis of a gemstone, it is sufficient to consider a normal view with respect to the gem’s table. Analysis of the Tolkowsky cut shows that the majority of light, 51%, comes from the medium angles, as does the fire. The facets of a stone that are brilliant have the potential to produce fire. Brilliance is a combination of lighted on and off facets. A stone that evenly returns light, with no dark facets, appears lacking in life. It is the distribution and number of dark and bright facets that produce brilliance in a gemstone, as shown in Fig. 4 (a). For comparison, Fig. 4 (b) shows fire as enhanced by a plurality of localized light sources.
The appearance of a gemstone depends in part on illumination conditions: diffuse illumination favors brilliance; localized illumination, such as spot lighting, favors fire. Regardless of the type of illumination, each illumination distribution has an associated angular spectrum; the product of the angular spectrums of the illumination and of the gemstone determines the stone’s appearance. One goal of optical design is therefore to increase the angular spectrum of the gem. In a sense, the multiple facets and their projections through internal reflections in a gemstone act like little windows that permit light to reach the observer. It is the distribution and variation in brightness from each of these windows that create contrast. The angles blocked by the observer’s head and by light leaking through the pavilion may help to create contrast. In the Tolkowsky cut, in fact, if the facets are aligned properly, the lack of the high angles blocked by the observer’s head produces eight arrowlike radial obscurations that enhance contrast. In a “nail-head” diamond, on the other hand, the observer’s head makes most of the table appear dark and unattractive.
Geometrical angular spectrum is a useful concept for understanding gem-stone appearance. For purposes of optical design, it allows the contributions of illumination conditions to be differentiated from the contributions of cut proportions. Angular spectrum analysis can be used to study fancy-shape cuts (a current pursuit of the American Gem Society). Figure 5, for example, shows a rendering of a heart cut and its integrated angular spectrum, characterized by an increased content of low angles as compared to a round brilliant gemstone.

Geometrical ray analysis of a gem-stone can be a complex matter because it involves nonsequential, polarization, splitting and birefringent ray tracing. It can be time consuming as well, given the large number of rays that need to be traced and graphically displayed. The gemstone industry uses basic and advanced programs such as GemCad8 and DiamCal9 to assist in calculating the precise weight and cut proportions of gemstones. Professional optical design software such as ASAP,10 FRED,11 LightTools,12 TRACEPRO13 and ZEMAX14 are capable of performing sophisticated analysis of gemstones. In some cases, however, in the analysis of gemstones custom programming may be required to properly display useful information.
The analysis of a gemstone involves several steps. First, a CAD file in DXF, IGES or another format that contains the gem geometry must be generated. This file is imported into an optical design program where sources and detectors are defined. Next, rays are traced and the ray coordinates and detector information are processed and displayed as needed. Figure 6 shows the rendering of a round brilliant diamond by FRED. Since the design and analysis of gems is not a standard practice, the optical designer faces the tasks of display construction and of defining metrics for gemstone performance. The optical design of gemstones is still in its infancy. With the advent of optimization algorithms in illumination optics, however, it is foreseeable that automatic optimization of gems will become a reality in the near future. When that time comes, it will be possible to select a material such as cubic zirconium, to choose a cut shape such as the princess and to optimize a cut to ensure the strongest brilliance, fire and scintillation. Historically, this work has been done though trial and error.
One useful criteria in the optical design of gemstones is the goal of bringing to the observer’s eye the maximum amount of light, while at the same time producing variations in light intensity to create contrast. To achieve this goal, light from the low, medium and high ray angles must be distributed evenly and thoroughly though the gem crown, table and bezel, so that the absence or presence of one of these angular ranges will contribute to creating contrast. In a superior cut gem, the blockage of the high angles to produce contrast. Light leakage through the pavilion is minimized. Fire is maximized by increasing ray deviation and minimizing leakage through the crown bezel.
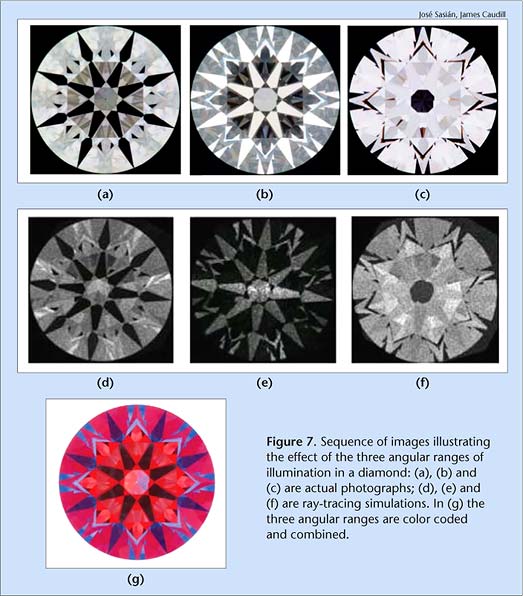
Figure 7 shows, in sequence, the appearance of a diamond as the low-, medium- and high-illumination ray angles are removed. The first row of images (a, b, and c) are actual photographs; the second row (d, e and f) are ray-tracing simulations. There is not complete agreement between the images because of a small error in acquiring the gem’s geometry as represented by the modeling abilities of the software package. Nevertheless, the images show how powerful ray tracing can be as a tool for the study of light behavior in gems. The colorful image in Fig. 7(g) is an actual photograph that for purposes of evaluation combines the low (blue), medium (red) and high (black) angles. Color hues are caused by the multiple ray refractions and by Fresnel ray splitting. This image shows a balanced distribution of each angular range in the gem crown so that light return and contrast are maximized to produce brilliance — the signature of a superior stone.
Gemstone fabrication and characterization technology have made important advances. Today, some cutters can produce stones in which each facet can be located to less than 0.01 mm and to 0.2°, a remarkable achievement. And variations on this order can indeed change a gemstone’s appearance. Sarin Technologies Ltd.16 produces a machine that is capable of acquiring gem proportions, facet angles and locations, and generating CAD files that can be imported into optical design and analysis programs.
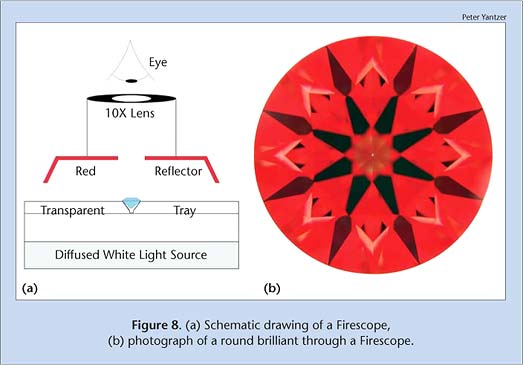
An elegant way to test a gem is the Firescope, invented in Japan in 1984.17 The Firescope is a simple device that uses a small cap, painted red on the inside, a diffused white-light source and a 10X eyepiece [see Fig. 8(a)]. The Firescope does not measure fire but instead shows the gem’s ability, per crown zone, to bring light to an observer’s eye [see Fig. 8(b)]. Under a Firescope, light leakage through the pavilion appears white and high angles appear black. Red or red hues indicate that the stone can bring different amounts of light from a given angular range. Both the spatial view of a gem and the angular spectrum are complementary representations. There is not a one-to-one mapping between these representations because, given Fresnel reflections, more than one ray direction can light a facet.
Progress in gemstone CAD modeling, precision facet cutting and optical characterization are bringing new excitement to the gemstone industry and to the optical design community. Thanks to these advances, jewelers are designing, enhancing and creating more beautiful gems. There are still many challenges to be met in areas including the automatic optical design of gems, grading, cutting and characterization, and understanding subtle details of the light- handling abilities of gems. From an optical designer’s point of view, the optical design of gem-stones is a refreshing and challenging endeavor.
